
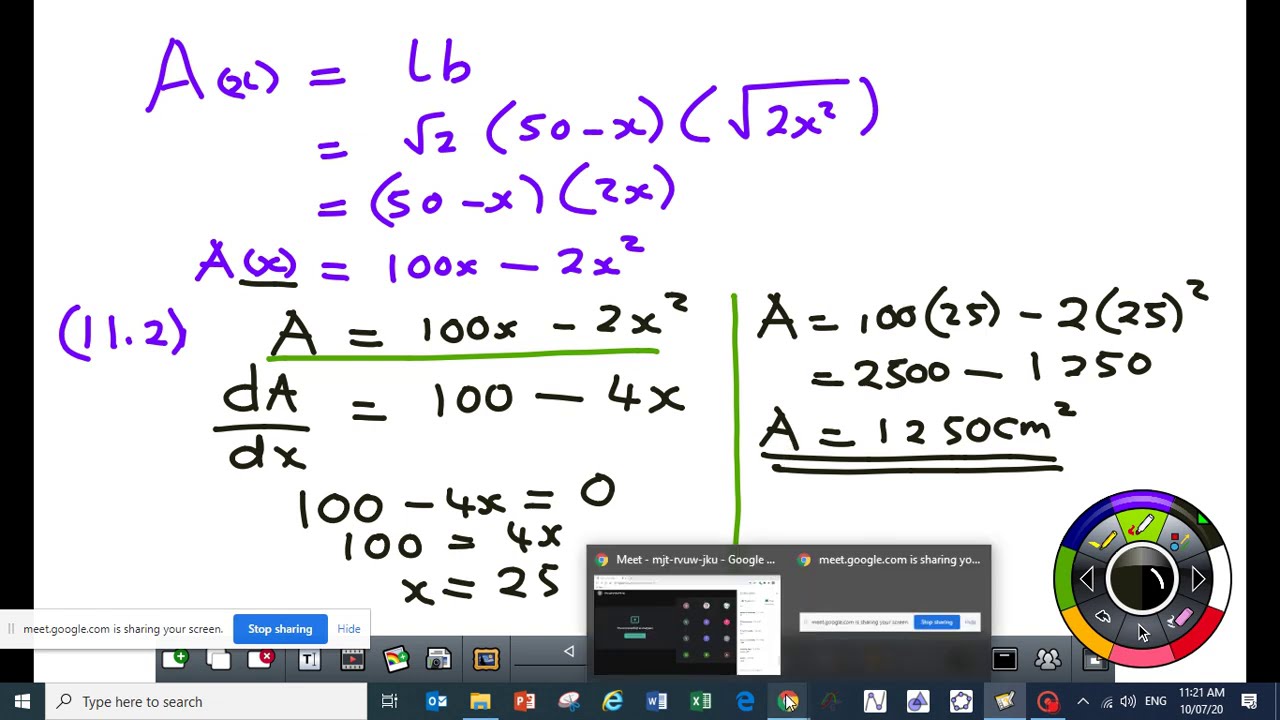
BASICS OF CALCULUS FOR BEGINNERS FULL
fans are currently raving about the new series of Full Metal Alchemist, especially as it is an almost direct adaptation of the manga, however in the light of all this new found glory, the original adaptation has become the topic of much debate and controversy, especially by those who once praised the show for being something. Or is it simply a case of people not seeing what they don't want to see, especially if there something new and shiny to watch? Isn't it strange then, that such a well known human trait can so easily be mistaken for something else entirely? What one does with their obsession though, well, therein lies an altogether different proposition, especially as people often define their "obsessions" in terms of what they love and hate, or even what brings them hope and fear. Obsession is a very strange thing indeed, and may be one of the few human traits that so clearly falls between heaven and hell.

However, the Elric brothers soon discover that there is more to the legendary stone than meets the eye, as they are led to the epicenter of a far darker battle than they could have ever imagined. Edward hopes to draw into the military's resources to find the fabled stone and restore his and Alphonse's bodies to normal. The fabled mythical object is rumored to be capable of amplifying an alchemist's abilities by leaps and bounds, thus allowing them to override the fundamental law of alchemy: to gain something, an alchemist must sacrifice something of equal value. With his supreme alchemy skills, Edward binds Alphonse's soul to a large suit of armor.Ī year later, Edward, now promoted to the fullmetal alchemist of the state, embarks on a journey with his younger brother to obtain the Philosopher's Stone.

We can then finally use a no-arbitrage argument to price a European call option via the derived Black-Scholes equation.Edward Elric, a young, brilliant alchemist, has lost much in his twelve-year life: when he and his brother Alphonse try to resurrect their dead mother through the forbidden act of human transmutation, Edward loses his brother as well as two of his limbs. In order to price our contingent claim, we will note that the price of the claim depends upon the asset price and that by clever construction of a portfolio of claims and assets, we will eliminate the stochastic components by cancellation. We will form a stochastic differential equation for this asset price movement and solve it to provide the path of the stock price. A geometric Brownian motion is used instead, where the logarithm of the stock price has stochastic behaviour. A standard Brownian motion cannot be used as a model here, since there is a non-zero probability of the price becoming negative. A vanilla equity, such as a stock, always has this property. For this we need to assume that our asset price will never be negative.

In the subsequent articles, we will utilise the theory of stochastic calculus to derive the Black-Scholes formula for a contingent claim. The derivative of a random variable has both a deterministic component and a random component, which is normally distributed. The fundamental difference between stochastic calculus and ordinary calculus is that stochastic calculus allows the derivative to have a random component determined by a Brownian motion. Ito's Lemma is a stochastic analogue of the chain rule of ordinary calculus. A fundamental tool of stochastic calculus, known as Ito's Lemma allows us to derive it in an alternative manner. The Binomial Model provides one means of deriving the Black-Scholes equation. This process is represented by a stochastic differential equation, which despite its name is in fact an integral equation.
The physical process of Brownian motion (in particular, a geometric Brownian motion) is used as a model of asset prices, via the Weiner Process. The main use of stochastic calculus in finance is through modeling the random motion of an asset price in the Black-Scholes model. In quantitative finance, the theory is known as Ito Calculus. Instead, a theory of integration is required where integral equations do not need the direct definition of derivative terms. This rules out differential equations that require the use of derivative terms, since they are unable to be defined on non-smooth functions. Many stochastic processes are based on functions which are continuous, but nowhere differentiable. Stochastic calculus is the area of mathematics that deals with processes containing a stochastic component and thus allows the modeling of random systems.


 0 kommentar(er)
0 kommentar(er)
